Tutorial: a Saturation Recovery Experiment
This hands-on-tutorial will teach you a method to process all kinds of relaxation times measurements and kinetic experimetnts too. We'll be working on a specific experiment of saturation recovery (please download it). You also need iNMR version 3.3.6 or later and Pro Fit (download the latter from QuantumSoft). You can substitute Pro Fit with another program of your choice. The trial version of Pro Fit is free, powerful and easy-to-use: we think it's the perfect choice. Our tutorial is based upon pro Fit trial 6.1.11.
If you have iNMR version 5.3.8, or later, you can work without auxiliary applications. This new version of iNMR can calculate the T1 with great accuracy, using a 3-parameters fit. The same algorithm can analyze both Saturation Recovery and Inversion Recovery experiments. You can still use Pro Fit, as described here, to get a more refined error analysis.
After unpacking the spectrum, you'll notice a file called “vdlist”. It is required by Bruker software and contains the list of variable delays. iNMR also needs the same list, yet under a different name and in a slightly simplified format. If you have a lot of experiments to process, you may find convenient to write a script for converting between the two formats. We'll do it manually instead. Duplicate the file vdlist. Rename the copy as “zeta”. Open it with a text editor (BBEdit is OK). The problem with this file is that not all the delays are expressed in the same unit. All you have to do is to change the first line from:
10minto
0.001Don't care about the missing s, iNMR ignores all kinds of units anyway. Now all the delays are expressed in seconds and this is enough. Save and close the file.
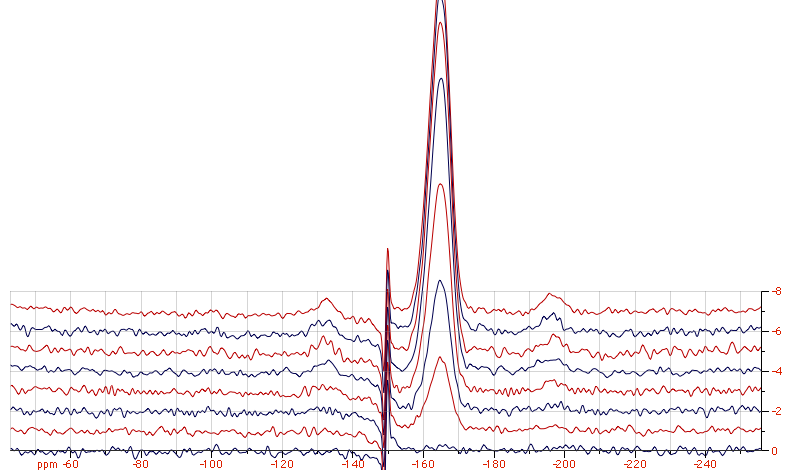
Open the spectrum with iNMR. The top row corresponds to the normal spectrum (fully relaxed), while the other rows contain the signal saturated at various degrees. For the sake of learning the different capabilities offered by iNMR we'll measure the intensities in two different ways. This will force us to spend more time than it's strictly necessary.
Integration is the first way. Extract the top row and define an integration range. Here are the step-by-step instructions if you have forgotten how to do it:
- alt-click above the top row
- choose File > Extract
- zoom in
- select the peak as shown below
- press the key i
- choose File > Close Extract
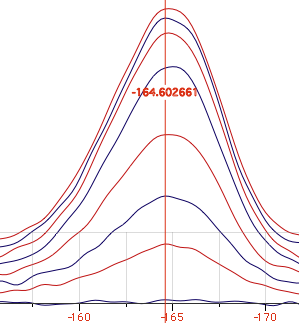
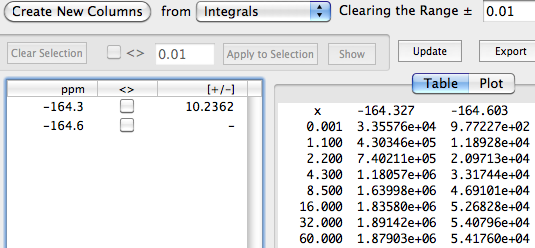
Choose Edit > Tabulator. Initially the table only contains the delays (column x). Press the button "Create New Columns". A new column will be created with all the integral values, because the menu selection is “integrals”. Select “Marks” from the same menu and click the button again. The third column will contain the values of height. The table on the left normally contains a list of peaks. In our case it contains the same peak measured in two different ways.
We can examine the difference between the two methods. The last integral (1.879) is smaller than the 7th (1.891). This is not good. We are inclined to choose the last column instead (peak heights). Click on “Plot” to verify that the build-up curves have the shape of an exponential. When you select the first entry (-164.3) you see the build-up of the integrals:
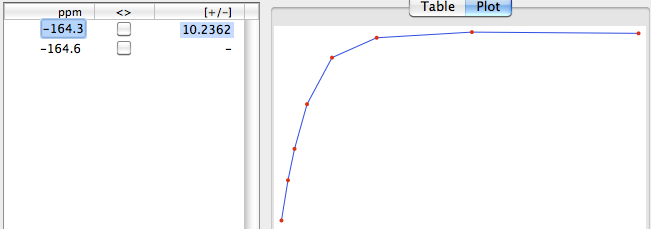
Why the first entry (into the small list) correpond to the integrals? You can recognize it by the the last column [+/-]. If it contains a value (like 10.2362), then iNMR calculates the integrals. The dash sign means: peak heights. You can edit these values; how the columns were created has no relevance at all. When you select the second entry (-164.6) you see the build-up of the peak heights:
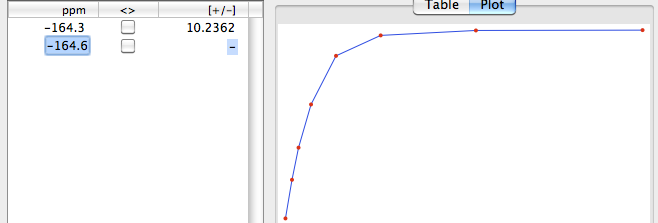
Click on the button “Export” to save the table as a file. Open the program “pro Fit trial” and import the same file with the command File > Import. When the window “Text file import options” appears, click on “Update”, then click OK. The table is imported:
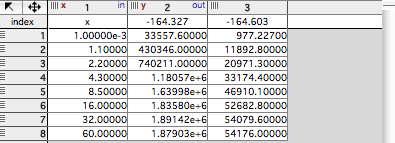
pro Fit, by default, fits data with a polynomial. Because this is not our case, choose Func > Exp. The new function shows 4 parameters (in bold face). They are too many. Click on x0 to make it a constant. Click again in the empty area to exit from the editing mode. Now x0 is written in normal font:

Now choose Calc > Fit from the menubar. The Fitting window appears. Our second column (the integrals) is selected by default. You can choose the other column, if you like, from the menu under “Output data”:
![]()
Click Fit to calculate the relaxation time. It corresponds to the parameter t0. Look at the Results window:
Chi squared = 1.0187e+9
Parameters: Standard deviations:
A = -1.8664e+6 ∆A = 1.3997e+4
x0 = 0.0000
t0 = 4.4247 ∆t0 = 8.5993e-2
const = 1.8898e+6 ∆const = 8688.4228
The equation is not specific to the saturation recovery experiment, therefore we get two independent parameters,
A and const. If we'd force the curve to start from zero, we'd reduce the degrees of freedom
of the problem and A
would be exactly the opposite of const. This is allowed by the program but not discussed here.
In conclusion the T1 = 4.4247s and its standard deviation = 0.086s.
The other curve is slightly less precise:
Chi squared = 1.1533e+6
Parameters: Standard deviations:
A = -5.3710e+4 ∆A = 469.9007
x0 = 0.0000
t0 = 4.5413 ∆t0 = 0.1034
const = 5.4318e+4 ∆const = 294.5254
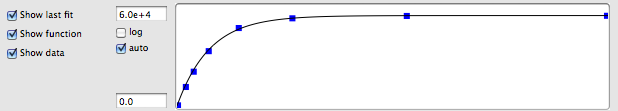
We have touched many important features of the iNMR tabulator. You can create how many columns you need, and they will usually point to different signals. Even if you have many relaxation times to measure into the same spectrum, you need to export just one table. Pro Fit will let you fit all the exponential curves sequentially, in almost no time. The results will all be conveniently collected into the same window. As you may realize, we have barely scratched the surface of pro Fit.
