iNMR icon

Simulate and Fit a Multiplet Under All Conditions

Not all spectra are well resolved. 2-D correlation spectra, for example, suffer from poor digital resolution (if compared to high-resolution 1-D spectra). It is not safe (and sometimes not even possible) to measure a coupling constant directly from such spectra, neither is reasonable to fit them with Lorentzian or Gaussian curves, because you normally pre-multiply the FID with a weighting function that imposes a different shape to the peaks. The solution is to simulate a multiplet in time domain and reprocess it with the same weighting function. A first-order multiplet is described by very few parameters, for this reason we don't need many experimental points (as we do with normal deconvolutions).

iNMR contains a module to simulate an isolated first-order multiplet subject to the same processing used for a given experimental spectrum. It is just one of four alternative and unrelated solutions:

  1. Create a simulation document to study second-order spectra or phenomena of exchange.
  2. Use the deconvolution module to disentangle overlapping signals in 1-D spectroscopy.
  3. Use the J manager for a simple, well resolved, 1-D spectrum.
  4. To extract a J from a 2-D spectrum, like a DQF-COSY, or from a badly-resolved spectrum, or when the shape strongly depends on processing, use the module described in this page.

We illustrate the example of a 2-D spectrum, but you can use the same tool with all kind of spectra (1-D to 3-D) of 1/2 spins. In 1-D cases, skip the step no. 4.

To Simulate and Fit a Multiplet Signal in a 2-D Spectrum:

Step 1

Process your spectrum starting from time domain. iNMR needs to find the parameters for the weighting functions. These parameters cannot be found if you start from an already processed spectrum (for example, with a different software).

Step 2

Choose the dimension to work along. The dialog “Format > Axes and Scales” shows which axis corresponds to which dimension. Normally the direct dimension (f-2) is better resolved and is richer in information content. Lay this dimension along the X axis (transpose the spectrum if necessary).

Step 3

Select a region around the multiplet with the mouse: the region becomes gray. Choose the region wide enough, because a surplus of points improves the accuracy of the fitting process.

Step 4

Select a row with alt-click. If there is room above the plot, the selected row will be reproduced there. Move the red mark up and down to select a different row. You would normally select the row where the signal is more intense. Skip this step in case of 1-D spectra or 1-D extracts.

Step 5

Choose the command Simulate > Multiplet. A new window will be created with a copy of the selected multiplet, taken from the selected row. This excerpt of the experimental spectrum is drawn as a black line. You will also see a red line, corresponding to a simulated multiplet. You can enlarge the window if you like.

Step 6

At the center of the window there is a blue reversed T. Drag it horizontally to change the central frequency of the red multiplet. Drag it vertically to move the plot up and down. If the experimental multiplet contains an antiphase coupling (that is, negative peaks) it will be necessary to center the plot vertically.

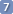
Step 7

Use the three menus at the top to introduce more couplings or change their multiplicity. Set the values of the coupling constants to create a mutual correspondence between experimental peaks and simulated peaks. It doesn't mind if their intensity is different, while it is important that each red peak overlaps with the corresponding black peak.

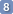
Step 8

Push the “Fit” button for an automatic refinement. iNMR will optimize frequency, width, intensity and the coupling constants and minimize the difference between the red and the black line (least squares principle).

You can use the other button (“Copy”) to store the coupling constants into the J Manager.

Related Topics

About Processing

Reducing the Number of Dimensions

Adding Projections around a 2-D Plot

Measurement of Long-Range H,C Couplings

 

Web Tutorial

Tutorial: How to Measure the Coupling Constants from a DQF-COSY